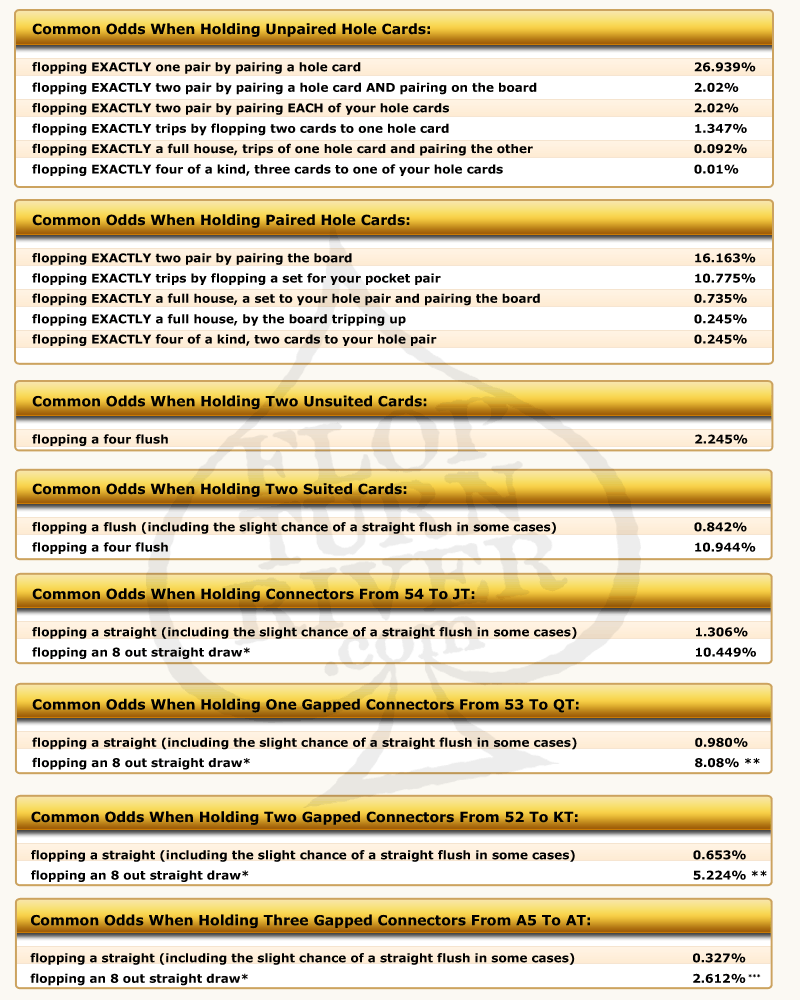
Lastly, the odds for the 8 out straight draws. For all the other odds, I have confirmed my results against reference material and they match. I have not found any references for these 8 out straight draws. I may have errors and I invite discussion.
The 5 card, 8 out straight draws fall into two categories. First, there is flopping 4 consecutive cards such as:
– A B C D –
So there are 4 outs to the left of A and 4 outs to the right of D.
We will call this a TYPE-1 8 out draw.
Secondly, there are double barreled gut shots (double bellied gut shots) such as:
A – C D E – G
So there are 4 outs to the left of C that will give you an A – E straight and there are 4 outs to the right of E that will give you a C – G straight.
We will call this a TYPE-2 8 out draw.
Holding connectors 54 through JT and flopping an 8 out draw.
First, we will compute the TYPE-1 draws. Given that our hole cards are F and G and they are connected, graphically here are the three TYPE-1 8 out draws:
D E F G – –
– E F G H –
– – F G H I
For each of these we are looking for 1 of the 4 left most flop cards, then 1 of the 4 right most flop cards, and then any card that DOES NOT give us a straight (there are 40). Then divide by the number of combinations. That gives you:
(4 * 4 * 40) / (50 * 49 *48) = 0.54422%
That is the chance of flopping the cards in exactly the order: L R x. There are 5 other combinations that have an equal chance:
L x R
R L x
R x L
x R L
x L R
So, the odds of flopping any one of those TYPE-1 draws would be:
6 * 0.54422% = 3.265%
In turn, there are 3 of those TYPE-1 draws, so the odds of flopping any of the TYPE-1 draws would be:
3 * 3.265% = 9.796%
Second, we will compute the TYPE-2 draws. Given that our hole cards are F and G and they are connected, graphically here are the two TYPE-2 8 out draws:
C – E F G – I –
– D – F G H – J
This turns out to be a problem where we are looking for a flop containing EXACTLY an X, Y and Z rank. We already computed that chance. It was S. There are two sets of X, Y and Z that would give us two TYPE-2 draws, so the chance is 2 * S or:
2 * 0.3265% = 0.653%
Lastly, the chance of any 8 out draw would be the sum of the chance of the TYPE-1 draws and the TYPE-2 draws. So,
9.796% + 0.653% = 10.449%
Holding one gapped connectors 53 through QT and flopping an 8 out draw.
First, we will compute the TYPE-1 draws. Given that our hole cards are F and H and they are one gapped, graphically here are the two TYPE-1 draws:
– – E F G H – – –
– – – F G H I – –
C D E F – H – – –
– – – F – H I J K
The first two require two cards to form up the 4 sequence. The second two require 3 cards to form up the sequence.
Using the numbers we figured out for the step above, that gives us:
2 * 3.265% + 2 * 0.3265 = 7.18%
Second, we will compute the TYPE-2 draws. Given that our hole cards are F and H and they are one gapped, graphically here are the three TYPE-2 draws:
B – D E F – H – – – –
– – D – F G H – J – – –
– – – – F – H I J – L
That would give us three TYPE-2 draws so the chance is 3 * S or:
3 * 0.3265% = 0.980%
Lastly, the chance of any 8 out draw would be the sum of the chance of the TYPE-1 draws and the TYPE-2 draws. So,
7.18% + 0.980% = 8.08% (I forgot to include two of the TYPE-1 draws in my initial posting. I have corrected the numbers.)
As a final note, the 53 and QT combinations cannot form one of the TYPE-2 draws as there is not enough room to the left and right respectively, so their odds are slightly lower.
Holding two gapped connectors 52 through KT and flopping an 8 out draw.
First, we will compute the TYPE-1 draws. Given that our hole cards are F and I and they are two gapped, graphically there are five TYPE-1 draws:
– – – F G H I – – –
– D E F H – I – – –
C D E F – – I – – –
– – – F – H I J K –
– – – F – – I J K L
The first requires two cards to form up the 4 sequence. The remaining 4 require 3 cards to form up the sequence.
Using the numbers we figured out for the step above that give us:
1 * 3.265% + 4 * 0.3265 = 4.571%
Second, we will compute the TYPE-2 draws. Given that our hole cards are F and I and they are two gapped, graphically here are the two TYPE-2 draws:
– C – E F G – I – – –
– – – – F – H I J – K –
That would give us two TYPE-2 draws so the chance is 2 * S or:
2 * 0.3265% = 0.653%
Lastly, the chance of any 8 out draw would be the sum of the chance of the TYPE-1 draws and the TYPE-2 draws. So,
4.571% + 0.653% = 5.224% (I forgot to include four of the TYPE-1 draws in my initial posting. I have corrected the numbers.)
As a final note, the 52 and KT combinations cannot form one of the TYPE-2 draws as there is not enough room to the left and right respectively so their odds are slightly lower.
Holding three gapped connectors A5 through AT and flopping an 8 out draw.
First, we will compute the TYPE-1 draws. Given that our hole cards are F and J and they are three gapped, graphically here are TYPE-1 draws:
C D E F – – – J – – –
– D E F G – – J – – –
– – E F G H – J – – –
– – – F – H I J K – –
– – – F – – I J K L –
– – – F – – – J K L M
These all require 3 cards to form up the sequence.
Using the numbers we figured out for the step above, that gives us:
6 * 0.3265 = 1.959%
Second, we will compute the TYPE-2 draws. Given that our hole cards are F and J and they are three gapped, graphically here are the two TYPE-2 draws:
D – F G H – J – –
– – F – H I J – L
That would give us two TYPE-2 draws so the chance is 2 * S or:
2 * 0.3265% = 0.653%
Lastly, the chance of any 8 out draw would be the sum of the chance of the TYPE-1 draws and the TYPE-2 draws. So,
1.959% + 0.653% = 2.612% (I forgot to include scads of the TYPE-1 draws in my initial posting. I have corrected the numbers.)
As a final note, the actual numbers vary all over the place for three gappers. Only the 95 and T6 three gappers can form all of the combinations listed. As the three gappers get closer to the edge, fewer combinations are possible. In short, do not expect much from three gappers.